I can’t seem to figure out how to connect a 3 way joint. I’m able to make a 90 degree by just using the follow me tool, however I’m not sure how to tie in the vertical and have a nice smooth outside corner. Please help!!
Extrude the top one half way through the first two, select all of the geometry, right click on it, choose Intersect Faces>With Selection and then erase what isn’t the 3-way joint.
I made it worse lol and have more questions now. I assumed that I needed to make a 10mm arc to make the horizontal portion of the joint since the outside radius of my pipe is 10mm, however I can’t seem to close the inside for some reason. I can’t figure out where I’m off. I would assume the outside corner would be a perfect miter from all three directions and would be the same whichever way you spin it… I apologize for being a dumb dumb
Apparently I couldn’t even manage to reply in the correct place either… I’m sorry for the trouble haha. If you could, please take a look at the last reply I had in this thread. As always I greatly appreciate your help!
The problem you are showing there is what’s known as the tiny face issue.
Sketchup struggles with very small tolerances, it wasn’t originally designed as software for 3d printing small things, it was for architectural stuff, so very small or very very large can cause problems.
What happens with very small things is that the vertices get so close together that sketchup things that are the same and fails to form the face between them. The tiny faces can exist but it can be difficult to create them.
So there have been a couple of ways of dealing with this depending on your workflow.
Originally people scaled up, edited the shape then scaled down again. This works but is a bit cumbersome, and you can end up ‘walking’ your model a long way from the origin.
Another option is to use a second instance of your component, scaled up and edited, the edits will be reflected in the small version, then the large one can be deleted. This is useful when you want to work on something small in the context of a larger model. As an example, you could make a copy of a door handle, move it outside your house and scale it larger than the house, detail it, the delete it and the original small one on the door inside the house will have the details without ever moving anywhere. This has colloquially become known as The Dave Method although he didn’t specifically invent it.
But realistically if you are designing for 3d printing there is no need for any of these geometry gymnastics. The .stl file format that you will export to print is unitless. Meaning it only knows it is a certain number of units large, those units can be anything. So, you can model in metres and export the stl then tell the printer it is millimetres. This can take a bit of fiddling with your export to understand what settings to export to suit your printer software, but that can be done with a simple cube of a specific size to understand the settings.
So having rambled on with all that, your model is failing because it is too small, work at a larger scale and the faces will form.
Here’s a simple method to go with all that. I haven’t used any specific dimensions and have made it quite thick so it is easier to see what is happening inside. But all the logic is there for an accurate pipe. One trick in there to help with cleanup is Weld Edges, otherwise you’ll end up with lots of bits of geometry to try and find to delete.
These types of tasks show the basic disadvantage of the SU - not handling real curves and converting them into segments. This causes errors like below. a quarter of a circle with the same radius was used to round off two lines. Depending on whether the straight lines came from the ends of the segments or from the centers, we get two different solids. In both cases we have equal segments and “equal” “arcs” - the same radius and the same angle.
From the above statement:
it is not always true
In this particular case, we have three equal cylinders that should intersect a sphere of the same diameter from three orthogonal x-y-z directions. Unfortunately, the “SU ball” in these three directions does not have the same shape .. Below, the same ball is superimposed on itself and rotated by 90 degrees in relation to the center. As you can see, the objects do not coincide. To prevent “wisdom” about “fight Z” I substract the spheres.
If we now add another difficulty, namely that the circles that are to be used to build the cylinders can be rotated relative to the center, we can see that this approach to the problem is a failure in advance, especially when the directions of the cylinders are diagonal!
So how do you “bite it”? This work only for orthogonal cylinders directions
1.Create 3 circles on the xyz axes
2. pull them out to the desired distance and cut their surfaces between them
3. use the selected arc and surface and the FollowMe tool to close the “corner”
4. Delete unnecessary geometry
5. Repeat steps 1-4 for the inside surface or scale the result to obtain the thickness of the connector
6. Tea and smile
No problem with small faces, scaling, golden methods … Unfortunately, Sketchup has flaws that can generate problems, but some people cannot see it.
Feel free to go and use another software since you find this one so difficult to use.
I am not embarrassed and I have been using it - for years because … imagine that there are areas where Sketchup is not fully true. But… feel free to call me ignorant or unteachable fools
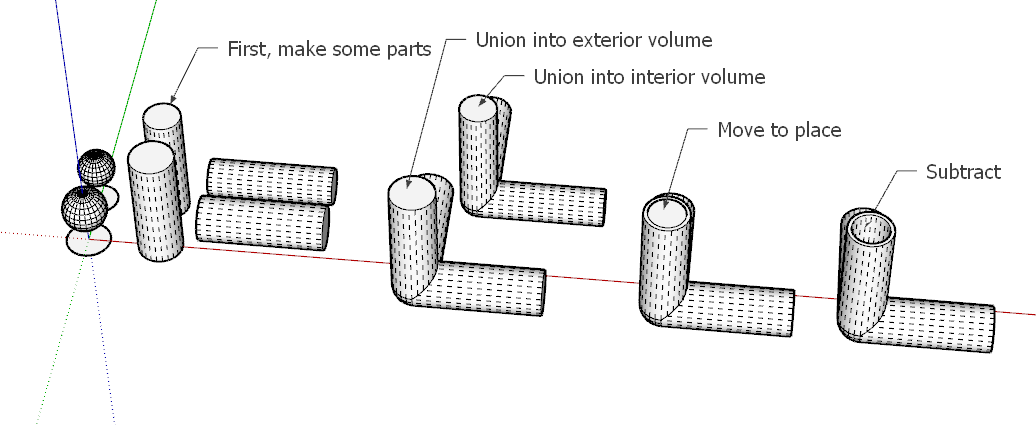
Another way of tackling this. Click in sequence on the scenes tabs of this SU file for ideas.
Three tubes junction.skp (329.3 KB)
I am working one one with all curves.
As promised. Click in sequence on the scenes tabs of this SU file. Anssi got the same idea of using a spherical part.
Three tube junction 2.skp (131.5 KB)
My version: Intersect Tubes Skill Builder
SketchUp Free does not have Solid tools
This is not much different from my proposal. The only difference is the corner filling. For me, the corner is built from the existing geometry, your solution allows you to incorrectly enter the diameter of the sphere.
I know. It can all be done with Intersect Faces, but it is more work.
Firstly, it is Aaron’s solution, secondly, it is a different type of connector.